Adam McBride's Thoughts on my newsletter Magic Square Puzzle
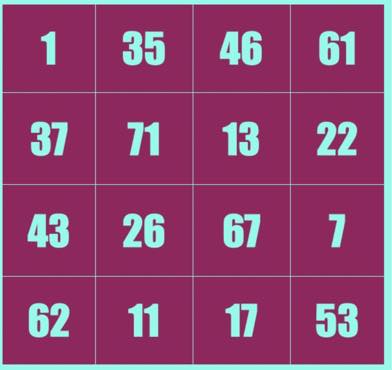
In my monthly newsletter for October 2021, I published the following Magic Square. It has the unusual property that if you square each number – it is STILL a magic square!
I was very pleased that Adam McBride, legendary mathematician and lecturer sent me some very comprehensive notes on the subject. They are reproduced below for your perusal.
Thanks Adam!
Some thoughts on your MAGIC square.
1. Because the diagonals do not produce the same sum as the rows and columns, the square is strictly a semi-magic square. However, its unusual extra property certainly makes it MAGIC in a different sense.
2. I wonder if any of your answers from students will mention that from the given array you can get infinitely many more with the same property in two ways.
Firstly it is easy to see that if every number in the array is multiplied by the same constant k, then the resulting array retains the MAGIC property. Indeed, if the common value of the row and column sums and of the sums of squares are s and t, respectively, then the corresponding common values for the new array are ks and k²t. Similarly, the MAGIC property is retained if every element is divided by the same non-zero constant. (The constant k need not even be an integer, but we usually restrict ourselves to that case to avoid fractions.)
Perhaps less obvious is the fact that the MAGIC property is retained if the same constant k is added to every element in the original array. With s, t as above the corresponding value for the sum in the new array is easily seen to be s+ 4k but a little bit of simple algebra is needed to show that t is replaced by t + 2ks + 4k². The same is true if we subtract k as we simply replace k by –k above.
3. As an illustration, suppose we subtract 36 from all the elements in the original array. The new array is
– 35 -1 10 25
1 35 -23 -14
7 -10 31 -29
26 -25 -19 17.
The original array has s = 143 and t = 7063.
The corresponding values for the new array are
-1 = 143 + 4 (-36) and 1951 = 7063 + 2.(-36).143 + 4. 36².
4. This reminds me of an amazing 8 x 8 magic square devised by Benjamin Franklin, the great American polymath and a Founding Father of the United States:
7 53 41 27 2 52 48 30
12 58 38 24 13 63 35 17
51 1 29 47 54 8 28 42
64 14 18 36 57 11 23 37
25 43 55 5 32 46 50 4
22 40 60 10 19 33 61 15
45 31 3 49 44 26 6 56
34 20 16 62 39 21 9 59 .
The common value of the row, column and diagonal sums is 260. If we replace each element by its square, so that 7 becomes 49, 53 becomes 2809 and so on, we obtain a new array which is also a magic square with row, column and diagonal sums equal to 11,180.
Not quite the same as yours but super-magic in a similar way.
