Are we Dividing Wrong?

So I’ve been thinking really hard for a few years now why it is that children seem to get so confused by division.

I’m not saying I have the full answer yet, but I do think that it might be a lot to do with the fact that for some reason we usually introduce division as sharing, before introducing the more powerful (and arguably simpler) concept of grouping.

RANT WARNING: I believe that both these labels are unhelpful, since both methods involve, um, sharing things into groups. Go figure.

The two are similar but different in one crucial respect. Specifically, in what we refer to as the sharing model, the divisor (the number after the ÷ sign) stands for the number of groups into which our original amount must be shared. In the grouping model, however, the divisor stands for the size of each individual group.

It gets worse further on in children’s experiences of division.

For example, when using a ‘bus stop’ or formal method to find 730÷5, we don’t say “OK, divide 7 into 5 equal groups”; we say “How many 5s are in 7 (hundred)?” In other words, we are specifying the size of a single group – 5.

Therefore, the whole formal methodology relies not on sharing but grouping. Yet by the time children meet grouping the idea of dividing as ‘cut this into this many parts‘ is so embedded that it is very difficult for them to make the conceptual leap.

In reality, sharing only works for very small (and whole number) divisors, but because it’s the first thing children learn, they revert back to it in panic when they meet harder division. (Think of a Y6 child drawing 12 circles when asked to divide something by 12 and proceeding to fill them with dots.)

Or, if asked to find 10÷1/2, they simply find half of ten. If they thought of it in terms of ‘how many halves in ten whole ones’ they would be more likely to come up with the correct answer of 20.

For this reason when introducing formal division layouts and methods, it may be very helpful to children to work with place value counters and cards such as in this example:

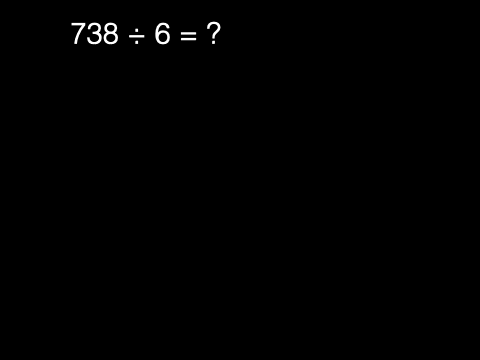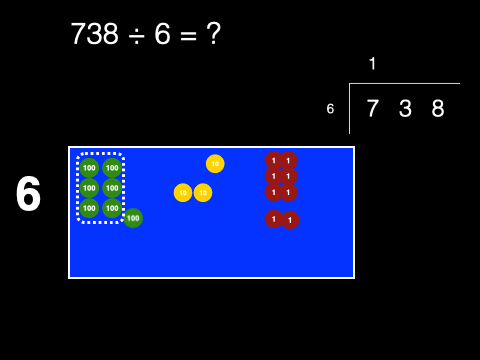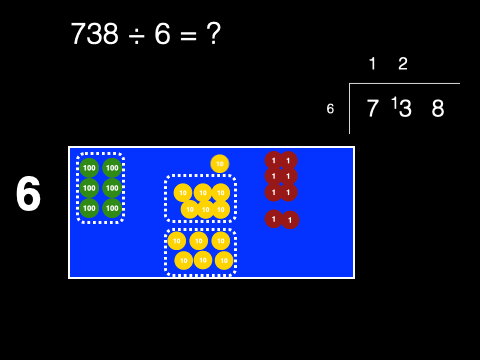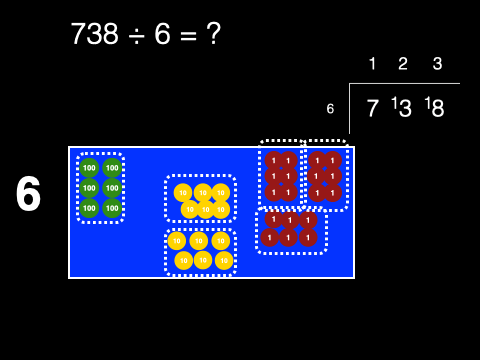
Ideally, children should work through this and similar problems, making groups of 6 counters, and splitting leftover counters into ten of whatever is in the next column, they mimic the written method. Although in reality, it is the written method that should mimic the action, not the other way round.

And sorry for reiterating it, but notice that at no point do we attempt to ‘cut things into 6 equal groups’…

So I’ve created this video aimed at addressing this, by suggesting we teach grouping BEFORE sharing. Controversial, I know.But there has to be a reason why division is so poorly understood – and if this helps even one child, I’m all for it.

P.S. I made this video in school time, but my generous HT has said I may share it with anyone who wants. It’s designed to be family-friendly in case you are emailing work home to parents at the moment.You can download the original from https://vimeo.com/504797544.